The Panknin Twist Formula
We met Dr. Walter Panknin at the 1989 Madison Area RC Society Symposium. His presentation at the MARCS Symposium, entitled "Flying Rainbows," included all of the fundamental information required to design and build a high performance swept wing tailless sailplane.
Copies of the 1989 Symposium Proceedings are no longer available through the Madison Area Radio Control Society. High resolution GIF and JPG images of the "Flying Rainbows" portion of the Proceedings are available from this link.
The formula presented below is important in that it provides, early in the design process, a well tested method of determining the geometric wing twist required for stable flight. The equations
There are six variables which influence the amount of required geometric wing twist:
-
taper ratio:
-
The greater the taper ratio, the more twist will be required. This is due to the loss of airfoil efficiency as the wing tip chord gets smaller.
-
aspect ratio:
-
The greater the aspect ratio, the less twist is required. However, as aspect ratio increases, and the wing chord gets proportionally smaller, it becomes more difficult to build a strong stiff wing.
-
sweep back angle:
-
The greater the sweep back angle, the less twist will be required. However, angles of sweep back in excess of 20 degrees adversely affect air flow over the wing and may lead to control problems.
-
airfoil moment coefficients:
-
The moment coefficient of the whole aircraft must be positive for stability. While some of this moment is derived from the downforce generated by the wing tips, it is also greatly influenced by the pitching moments of the airfoils used. Sections used on contemporary swept wing tailless aircraft have near zero pitching moments.
-
desired static stability value:
-
Increased static stability (CG further ahead of the neutral point) dictates increased wing twist.
-
design coefficient of lift:
-
The greater the design coefficient of lift, the more wing twist will be required. The design coefficient of lift should be the coefficient of lift at cruise speed so that trim drag is minimized. Some amount of up trim is expected to be necessary for thermalling.
-
airfoil zero lift angles:
-
The zero lift angles of the root and tip airfoils influence the geometric wing twist required.
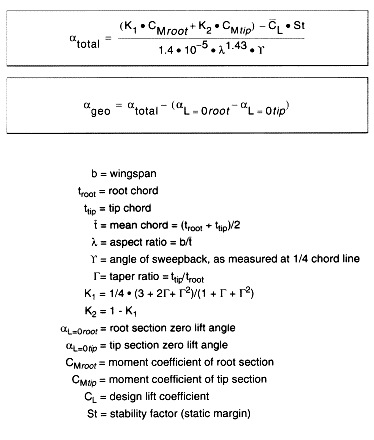
Calculation of required wing twist using the above formulae can be easily accomplished on a hand-held calculator. If you have a computer and Microsoft QuickBASIC, you might want to download the documentation and programs from the links below.
A sample planform, and computation of required geometric wing twist...
Span = 120 inches; root chord = 13 inches; tip chord = 11 inches; sweep angle = 17 degrees, as measured at the quarter chord line.
Root section E 222, zero lift angle = -3.65 degrees, pitching moment = -0.097. Tip section E 230, zero lift angle = +1.73, pitching moment = +0.053.
Design lift coefficient = 0.6; stability = 0.05
The taper ratio is 11/13 = 0.85; the average (mean) chord is (13 + 11)/2 = 12; the aspect ratio is 120/12 = 10.
K1 turns out to be 0.52; K2 equals 0.48.
The main formula shows that a total of -8.6 degrees is required for this planform. Once aerodynamic twist (due to the zero lift angles of the two sections) is accounted for, it is found that roughly 3.2 degrees of geometric washout is required.
Applications and spreadsheets
-
With the assistance of Alan Halleck, we've written QuickBASIC applications for both the Macintosh and IBM-compatible operating systems. The text files identified by the links below each contain documentation and two programs, one for saving section aerodynamic data to disk and the other for computing the wing twist, CG location, and other variables. Set your web browser to save the appropriate link as a text file. You can then open the saved text file with a text editor. The coding for the two programs needs to be extracted and resaved within separate files. After you've read the documentation and know how the two programs work, use QuickBASIC to read and run the individual applications.
MAC; the Panknin twist program, the disk files creator application, and documentation for the Macintosh.
IBM-compatible ; the Panknin twist program, the disk files creator application, and documentation for IBM compatibles.
-
Joa Harrison has written an Excel/Appleworks spreadsheet template which includes all of the computations performed by the QuickBASIC applications.
twist2.xls.zip; the twist2 template for Excel.
twist2.cwk.sit; the twist2 template for Appleworks.
-
Glyn Fonteneau modified Joa's spreadsheet to include winglet dimensions, based on information in Nickel and Wohlfahrt's "Tailless Aircraft in Theory and Practice."
twist3.xls.zip; the twist3 template for Excel.
twist3.cwk.sit; the twist3 template for Appleworks.
Final notes
No matter the resulting planform of your design, always keep the following five rules in mind:
-
Understand what you do
-
Build stiff, not just strong
-
There can be NO play in the linkages
-
Be sure of the precise location of the CG
-
Have confidence that it will fly
The complete text of Dr. Panknin's address, along with the full complement of diagrams, is available within the Proceedings of the 1989 MARCS Symposium. Contact Al Scidmore, 5013 Dorset Drive, Madison WI 53711 USA for ordering information.
links page for conventional aircraft
links page for tailless aircraft
plotters and helpers main page
B2Streamlines home page.

